My research is lies at the intersection between applied math and condensed matter physics with a particular focus on electronic properties of materials. Most recently, I’ve been interested in understanding electron-electron interactions in moiré materials (in particular twisted bilayer graphene). Previously, I worked on sparse basis construction for insulating materials. A list of my publications is below.
Moiré Materials
When two periodic patterns are twisted relative to each other, a large scale pattern, called a moiré pattern, is formed. Recently, it was discovered that by placing two thin sheets of a material on top of each other with a relative twist, the interplay between the small scale atomic configuration and the large scale moiré pattern leads to remarkable behaviors such as correlated insulators and superconductivity. These behaviors are due in part to the fact that the interactions between the two scales (atomic and moiré) can suppress the kinetic energy of the electrons making electron-electron repulsion dominant. In my work, I have studied the role of electron-electron repulsion in twisted bilayer graphene and its implications for the ground states.
Sparse Basis Construction
 Given a subspace of a vector space, a standard step in most algorithms is to construct an orthogonal basis for this subspace. Not all orthogonal bases are equally well suited for computation however.
For studying systems with local interactions, bases which decay quickly in space lead to sparse equations which drastically speed up calculation.
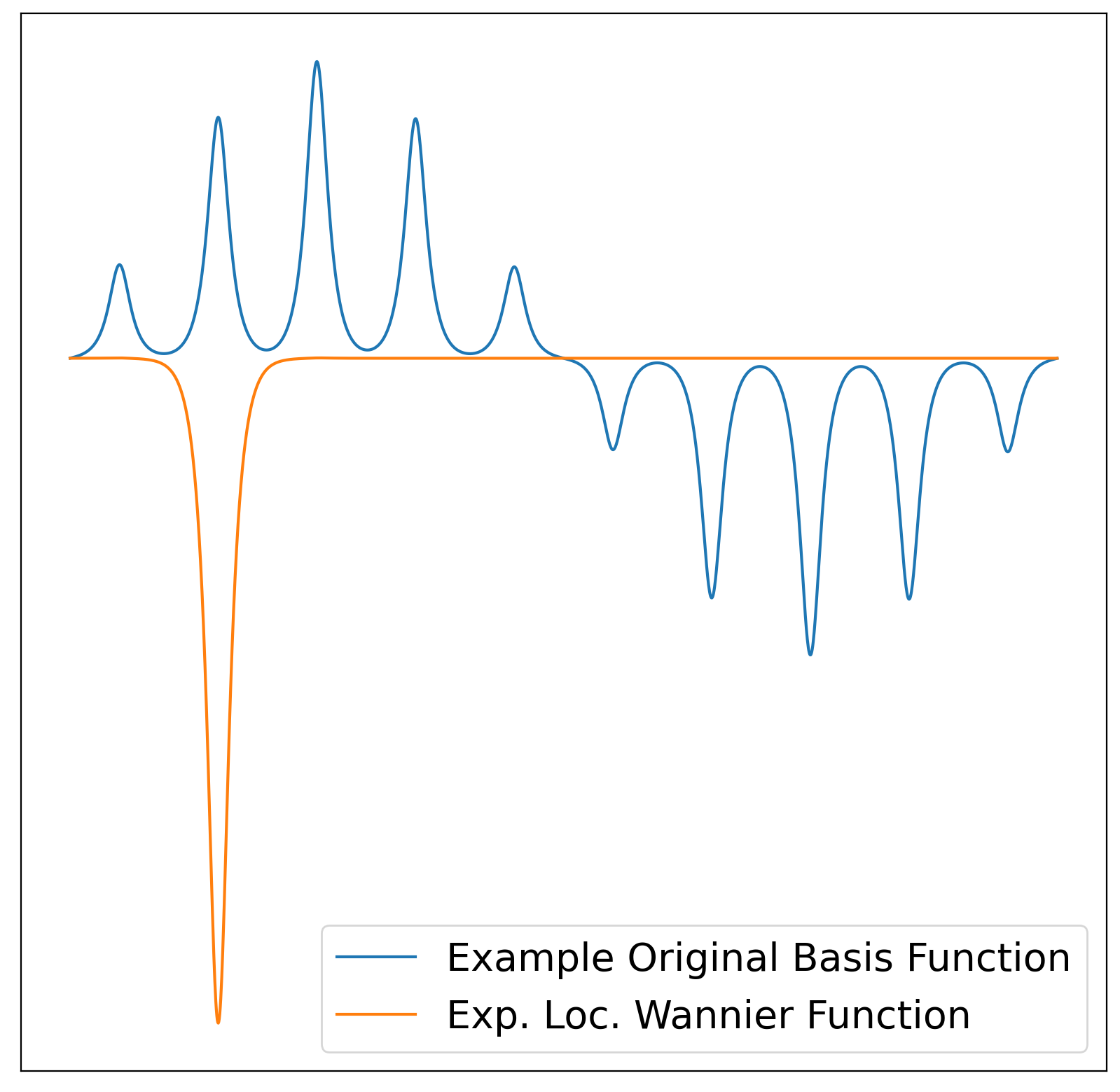
In electron structure theory, orthogonal bases which decay exponentially quickly in space are called exponentially localized (generalized) Wannier functions (ELWFs). In my work, I proposed an algorithm for constructing ELWFs and proved its correctness for a large class of subspaces (Left: A low energy eigenfunction of -Δ + V and an ELWF which is contained in the low energy eigenspace).
Given a subspace of a vector space, a standard step in most algorithms is to construct an orthogonal basis for this subspace. Not all orthogonal bases are equally well suited for computation however.
For studying systems with local interactions, bases which decay quickly in space lead to sparse equations which drastically speed up calculation.
In electron structure theory, orthogonal bases which decay exponentially quickly in space are called exponentially localized (generalized) Wannier functions (ELWFs). In my work, I proposed an algorithm for constructing ELWFs and proved its correctness for a large class of subspaces (Left: A low energy eigenfunction of -Δ + V and an ELWF which is contained in the low energy eigenspace).
Publications
| [18] | J. Basso, T. Bergamaschi, L. Lin, M. Ragone, and K. D. Stubbs, “DFT Based Ab Initio Modeling of Twisted Bilayer Graphene,“ in preparation, 2025. | |
| [17] | R. Kim, W. Kim, K. Stubbs, S. Louie, L. Lin, “DFT Based Ab Initio Modeling of Twisted Bilayer Graphene,“ in preparation, 2025. | |
| [16] | K. D. Stubbs, T. Kong, A. B. Watson, M. Luskin, “Metallic Ground States in interacting twisted bilayer graphene with realistic relxation,“ in preparation, 2025. | |
| [15] | K. D. Stubbs, M. Ragone, A. H. MacDonald, and L. Lin, “The Many-Body Ground State Manifold of Flat Band Interacting Hamiltonian for Magic Angle Twisted Bilayer Graphene,“ arXiv preprint arXiv:2503.20060, 2025. | link |
| [14] | K. D. Stubbs, S. Becker, and L. Lin, “On the Hartree–Fock ground state manifold in magic angle twisted graphene systems,” Multiscale Modeling & Simulation, vol. 23, no. 3, 2025. | link |
| [13] | T. Kong, A. B. Watson, M. Luskin, and K. D. Stubbs, “Interacting twisted bilayer graphene with systematic modeling of structural relaxation,” Electronic Structure, vol. 7, no. 3, 2025. | link |
| [12] | S. Becker, L. Lin, and K. D. Stubbs, “Exact Ground State of Interacting Electrons in Magic Angle Graphene,” Communications in Mathematical Physics, vol. 406, no. 6, 2025. | link |
| [11] | A. Alase, K. D. Stubbs, B. C. Sanders, and D. L. Feder, “Erasure conversion in Majorana qubits via local quasiparticle detection,” Physical Review Research, vol. 6, no. 4, 2024. | link |
| [10] | J. Lu and K. D. Stubbs, “Algebraic localization of wannier functions implies chern triviality in non-periodic insulators,” in Annales Henri Poincaré, Springer, 2024. | link |
| [9] | F. M. Faulstich et al., “Interacting models for twisted bilayer graphene: A quantum chemistry approach,” Physical Review B, vol. 107, no. 23, 2023. | link |
| [8] | J. Lu, K. D. Stubbs, and A. B. Watson, “Existence and computation of generalized wannier functions for non-periodic systems in two dimensions and higher,” Archive for Rational Mechanics and Analysis, 2022. | link |
| [7] | S. Brandsen, K. D. Stubbs, and H. D. Pfister, “Reinforcement Learning with Neural Networks for Quantum Multiple Hypothesis Testing,” Quantum, vol. 6, 2022. | link |
| [6] | K. D. Stubbs, A. B. Watson, and J. Lu, “Iterated projected position algorithm for constructing exponentially localized generalized wannier functions for periodic and nonperiodic insulators in two dimensions and higher,” Physical Review B, vol. 103, no. 7, 2021. | link |
| [5] | J. Lu and K. D. Stubbs, “Algebraic localization implies exponential localization in non-periodic insulators,” arXiv preprint arXiv:2101.02626, 2021. | link |
| [4] | A. N. Morozovska, E. A. Eliseev, K. D. Stubbs, R. Vasudevan, Y. Kim, and S. V. Kalinin, “Phase diagrams of single-layer two-dimensional transition metal dichalcogenides: Landau theory,” Physical Review B, vol. 101, no. 19, 2020. | link |
| [3] | S. Brandsen, M. Lian, K. D. Stubbs, N. Rengaswamy, and H. D. Pfister, “Adaptive procedures for discriminating between arbitrary tensor-product quantum states,” in 2020 IEEE International Symposium on Information Theory (ISIT), IEEE, 2020. | link |
| [2] | Z. Cai, J. Lu, and K. D. Stubbs, “On discrete wigner transforms,” arXiv preprint arXiv:1802.05834, 2018. | link |
| [1] | W. Czaja, B. Manning, J. M. Murphy, and K. D. Stubbs, “Discrete directional gabor frames,” Applied and Computational Harmonic Analysis, vol. 45, no. 1, 2018. | link |